Can you quickly and easily tell how intelligent someone is? There are some obvious ways to determine if someone is highly intelligent, like when you see them work out a complex trigonometry problem on a blackboard or when they can easily explain the science behind mRNA vaccines or dark matter.
But there are also those we meet at social gatherings who immediately make us think they are very smart. Usually, it isn’t because they are making a long-winded speech about the fall of the Roman Empire or explaining quarks. We know they are intelligent because of the way they interact with people and ideas.
A Redditor named SomethingAbout2020 asked people on the AskReddit forum to share the “non-obvious signs” that people are intelligent. Many of their responses centered on highly intelligent people being open-minded, curious, and not wasting their time arguing with others.
Brilliant people are confident in what they know, consider other people’s opinions, and readily admit when they don’t know the answer.
What are 15 'non-obvious' signs that someone is really smart?
1. They know what they don't know
"They acknowledge areas where they lack knowledge."
"'Never pretend to know something when you don't' is something I always teach. It covers lying and ignorance."
2. They consider other people's ideas
"They’ll listen to the other's facts and points and take them into account when giving an objection."
"One of the best developers at my last job and manager of a project I was at is an extremely intelligent person. ... One thing I noticed is how he would take everyone's opinion into account. He would take my opinions into consideration even if I'm not a smarter person or know less about development."
3. They make you feel smart
"Talking to a dumb person will make you feel smart. Talking to a smart person will make you feel dumb. Talking to a very smart person will make you feel smart."
4. They see patterns
"Part of the reason smart people throughout history are well-known is because they discovered something new and figured out how to maximize its potential. Darwin was a guy who discovered a bunch of islands with slightly different animals. He then collected and analyzed that data to come up with the theory of evolution, which was largely correct. Einstein’s theory of relativity was based off of his observation that physics acted on everything equally. He figured out that “exceptions” were because of the way high-speed objects interact with the universe’s speed limit (the speed of light). He recognized these exceptions by gathering them and recognizing the pattern between them all, then created his theory of relativity based on that."
 A painter making a mural. via Canva/Photos
A painter making a mural. via Canva/Photos
5. They consider multiple intelligences
"They realize not everyone is smart the same way. Your 'stupid hick neighbor' might have dropped out of school in 8th grade, but he can drive your car once and tell you exactly what's wrong, then fix it. That a**hole in school that had no empathy for anyone and showed no emotion made that sci-fi sh*t you thought would never be real. Yeah, she's dingy and her worldview is tiny, but she's the best teacher you've ever met and inspires tons of kids to go on and do great things with themselves. There's no one-size-fits-all answer here, really."
People who are super bright are probably familiar with Howard Gardner's theory of multiple intelligences. The theory suggests that people have more than just one type of just one type of intelligence, like being good at mathematics. Gardener says there are several, including musical, spatial, linguistic, interpersonal, intrapersonal, and kinesthetic intelligence. This theory allows people to appreciate different forms of intelligence that may not be of the academic variety.
6. They choose their battles
"When another person is not able to process something and, therefore, sticks with his opinion, after a few tries, the smart person just gives up. There is no use in trying to make someone understand something while they already have an uneducated opinion."
7. They speak to their audience
"They know how to explain concepts on just about any level, tailoring that level to their intended audience, and without coming across as condescending in any way."
"I heard a saying that went 'you have to be an expert to explain it simply.'"
8. They're confident in their intelligence
"Not constantly bragging about their intelligence. If they truly are smart, people can figure that out pretty quickly without them doing anything to show it."
"You generally only brag about things you're insecure about because you seek validation. If you are very comfortable with your intelligence, then you may not care if someone misinterprets you and makes you look dumb or something. You have nothing to prove. That's not just for intelligence but for anything."
9. They're funny
"I think the smart people are even more funny than stupid people because smart people understand the complexity behind humor and can make their jokes reflect that."
Scientific studies show that funny people, especially those with a dark sense of humor, are more intelligent than their not-so-funny peers. Researchers argue that it takes cognitive and emotional ability to make people laugh, and analysis shows that funny people have higher verbal and non-verbal intelligence.
 A man laughing at his friend's joke.via Canva/Photos
A man laughing at his friend's joke.via Canva/Photos
10. They mind their own business
"This is a big one. They keep to themselves and deal with their own drama."
11. They aren't necessarily great students
"Believe it or not 'average' or 'above average' students are often smarter than those with straight A’s on the report cards. They do enough to pass well and get what they want but don’t let the academic system control them. Life isn’t all about booksmarts. This shows they are independent thinkers and don’t get wrapped up in designed systems. Not all, but many. Many kids who are forced to always be exceptional in school can end up the worst off and can develop deeper issues."
12. They are good listeners
"They actually listen to who they are talking to as opposed to waiting for their turn to talk."
13. Curiosity
"It really does seem to be one of the single greatest differentiators between average and smart."
A meta-analysis of over 50,000 students from around 200 separate studies found that students who are curious do better in their school work over those who are not. Why is curiosity so important when it comes to IQ? “Curiosity is basically a hunger for exploration. If you’re intellectually curious, you’ll go home, you’ll read the books," Dr Sophie von Stumm, the study’s first author, said. "If you’re perceptually curious, you might go traveling to foreign countries and try different foods.”
14. Comfortable in silence
"Being comfortable enough to allow a moment of complete silence while you think when the natural instinct of most is to immediately start replying tells me that you are, at the very least, mindful of what you want to say."
15. Unattached to their opinions
"Most of the smart people I know are not pushy with their opinions; by contrast, most of the opinionated people I know are flaming morons. I don't know if there's a correlation there, but my anecdotal experience has always been that the more eager someone is to state their opinion, the less that opinion is probably worth."
This article originally appeared last year. It has been updated.


 Sandra’s granddaughter, E’s daughter, and another friend at an indoor park (July 2025)
Sandra’s granddaughter, E’s daughter, and another friend at an indoor park (July 2025) One of the ladies Sandra sponsored from Venezuela and her partner during Sandra’s first visit to meet her (December 2023)
One of the ladies Sandra sponsored from Venezuela and her partner during Sandra’s first visit to meet her (December 2023) A man handing a woman his business card at an event.via
A man handing a woman his business card at an event.via  A man and woman sharing a drink at a bar.via
A man and woman sharing a drink at a bar.via 
 A painter making a mural. via
A painter making a mural. via
 A man laughing at his friend's joke.via
A man laughing at his friend's joke.via Text Phone GIF by Life at Lower
Text Phone GIF by Life at Lower 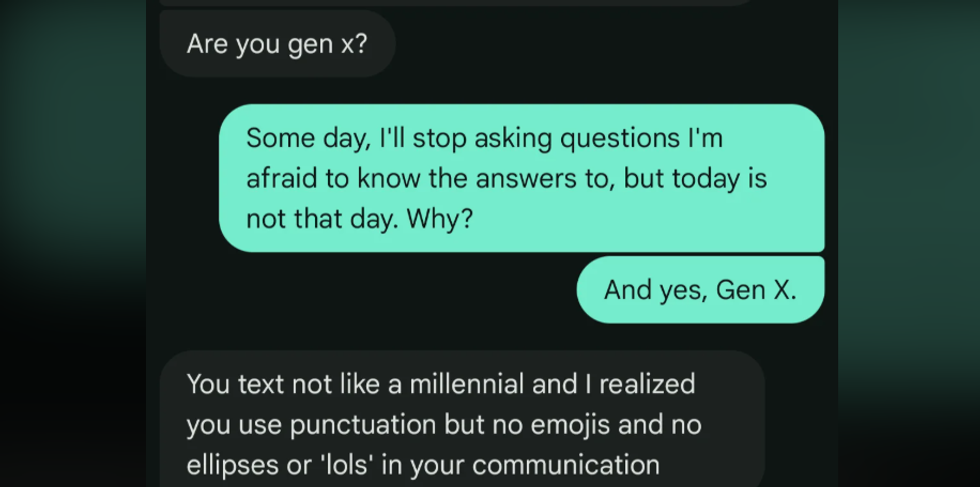 Gen Xer shares text convo with Millennial.Image via Reddit/Kestrel_Iolani
Gen Xer shares text convo with Millennial.Image via Reddit/Kestrel_Iolani steve buscemi youth GIF
steve buscemi youth GIF  Beauty Looking GIF
Beauty Looking GIF  80S Hair Flip GIF Giphy
80S Hair Flip GIF Giphy  1980s tv GIF by absurdnoise
1980s tv GIF by absurdnoise